Engineering Software
Physical Properties
This section provides free Physical Properties.
Physical Properties section covers the following area:
Single Species Approach
Physical Properties Single Species Approach
Introduction
This section provides a Physical Properties analysis for single species.
Analysis
In the presented Physical Properties analysis, only ten (10) basic species are considered behaving as an ideal gas -- ideal gas state equation is valid -- pv = RT.
For each reaction species, the thermodynamic functions specific heat, specific enthalpy and specific entropy as functions of temperature are given in the form of least squares coefficients as follows:
Cp/R = A1 + A2T + A3T^2 + A4T^3 + A5T^4
H/(R*T) = A1 + A2T/2 + A3T^2/3 + A4T^3/4 + A5T^4/5 + A6/T
S/R = A1lnT + A2T + A3T^2/2 + A4T^3/3 + A5T^4/4 + A7
or
S/R = A1lnT + A2T + A3T^2/2 + A4T^3/3 + A5T^4/4 + A7 - lnp
For each species, two sets of coefficients are included for two adjacent temperature intervals, 273 to 1,000 [K] and 1,000 to 5,000 [K]. The data have been constrained to be equal at 1,000 [K].
For example, physical properties for both reactants and combustion products are very important and need to be known in order to carry out successful combustion calculations.
The plot in Figure 1 depicts how the species specific enthalpy values change with the temperature. The physical properties provided in this plot come from the JANAF Thermochemical Data - Tables, 1970.
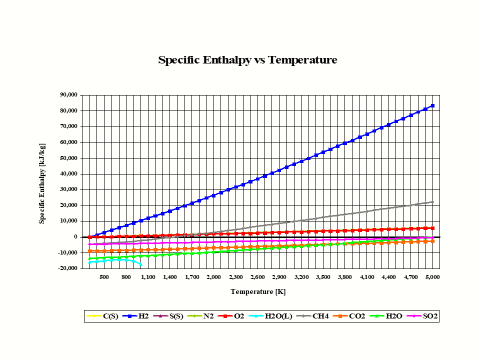
Figure 1 - Enthalpy vs Temperature
In general, specific enthapy values increase with an increase in temperature.
It is interesting to note that the specific enthalpy value for basic combustion elements such as carbon (C), hydrogen (H2), sulfur (S), oxygen (O2) and nitrogen (N2) is equal to zero at the standard combustion conditions of 298 [K] and 1 [atm].
Also, it should be mentioned that for ideal gas species, the specific enthalpy values are only dependent on the temperature.
Assumptions
Considered species behave as an ideal gas.
Governing Equations
For each reaction species, the thermodynamic functions specific heat, specific enthalpy and specific entropy as functions of temperature are given in the form of least squares coefficients as follows:
Cp/R = A1 + A2T + A3T^2 + A4T^3 + A5T^4
H/(R*T) = A1 + A2T/2 + A3T^2/3 + A4T^3/4 + A5T^4/5 + A6/T
S/R = A1lnT + A2T + A3T^2/2 + A4T^3/3 + A5T^4/4 + A7
or
S/R = A1lnT + A2T + A3T^2/2 + A4T^3/3 + A5T^4/4 + A7 - lnp
For each species, two sets of coefficients are included for two adjacent temperature intervals, 273 to 1,000 [K] and 1,000 to 5,000 [K]. The data have been constrained to be equal at 1,000 [K].
Also,
U = H - p*v*MW or U = H - R*T
G = H - S*T
and
u = h - p*v or u = h - R*T/MW
g = h - s*T
Legend:
Cp -- Specific Heat [kJ/kmol*K]
cp -- Specific Heat [kJ/kg*K]
MW -- Molecular Weight [kg/kmol]
R -- Universal Gas Constant [kJ/kmol*K]
Gas Constant = R/MW [kJ/kg*K]
H -- Specific Enthalpy [kJ/kmol]
h -- Specific Enthalpy [kJ/kg]
T -- Temperature [K]
S -- Specific Entropy [kJ/kmol*K]
s -- Specific Entropy [kJ/kg*K]
p -- Pressure [atm]
U -- Internal Specific Energy [kJ/kmol]
u -- Internal Specific Energy [kJ/kg]
v -- Specific Volume [m^3/kg]
G -- Gibbs Free Specific Energy [kJ/kmol]
g -- Gibbs Free Specific Energy [kJ/kg]
Conclusions
In general, specific enthalpy values increase with an increase in temperature.
It is interesting to note that the specific enthalpy value for basic combustion elements such as carbon (C), hydrogen (H2), sulfur (S), oxygen (O2) and nitrogen (N2) is equal to zero at the standard combustion conditions of 298 [K] and 1 [atm].
Also, it should be mentioned that for ideal gas species, the specific enthalpy values are only dependent on the temperature.
References
JANAF Thermochemical Data - Tables, 1970